Trending Content
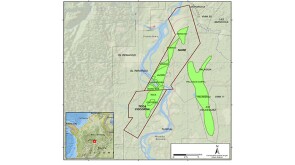
Kampala is drawing now on international experience to sidestep the “oil curse” before first crude flows from its Lake Albert developments.
The supermajor expects its latest development will be online by 2027 and add 250,000 B/D to Guyana's soaring output.
Independent producer divests interest in Buckskin field along with stakes in a pair of LLOG-led discoveries.
-
The new vessels bring QatarEnergy’s fleet to 104 as the company ramps up production with its North Field expansion.
-
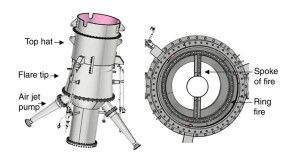
The US supermajor aims to speed the commercialization of a new liquid solvent that strips carbon dioxide from industrial flue gas.
-
Samsung E&A takes home $6 billion of the total amount as EPC contractor.
Get JPT articles in your LinkedIn feed and stay current with oil and gas news and technology.
-
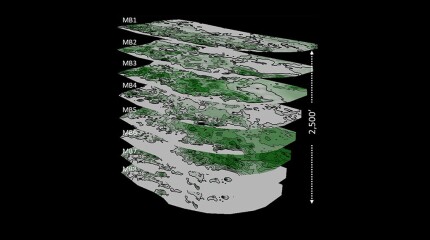
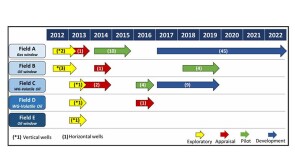
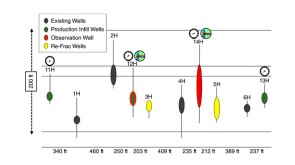
A 44-well development tests what ConocoPhillips has learned about maximizing the value of the wells by figuring out how they drain the reservoir.
-
The use of oil-based muds has precluded countless drill cuttings from being used to predict reservoir fluids despite once being part of the reservoir. A 6-decade-old technology may be on the cusp of changing that.
-
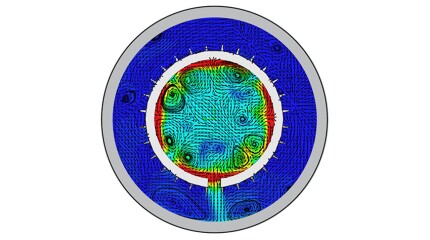
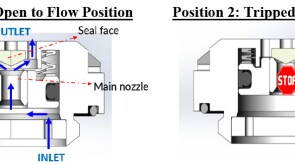
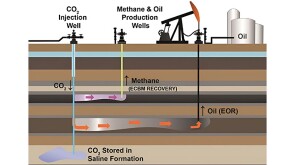
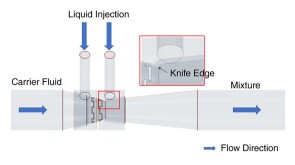
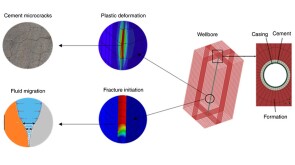
To avoid costly interventions like sidetracking or wellbore abandonment, a check-valve system was installed near the sandface within three injector wells which prevented the mobilization of fines from the reservoir into the wellbore by stopping backflow.
-
The challenges that geothermal energy faces to become a leading player in the net-zero world are well within the areas of expertise of the SPE community, ranging from rapid technology implementation and learning-by-doing to assure competitiveness to establishing suitable funding mechanisms to secure access to capital.
Access to JPT Digital/PDF/Issue
Sign Up for JPT Newsletters
Sign up for the JPT weekly newsletter.
Sign up for the JPT Unconventional Insights monthly newsletter.
President's Column
-
SPE President Terry Palisch is joined by Paige McCown, SPE senior manager of communication and energy education, to discuss how members can improve the industry’s public image.
-
SponsoredExpanding its portfolio of high-tier technologies, TAQA develops a high-performance perforation-plugging patented product.
-
SponsoredWith almost three-quarters of the global greenhouse gas emissions coming from the energy sector, there is a heavy burden and a huge responsibility on the shoulders of all countries of the world to transform the energy sector to be cleaner and greener by eliminating these emissions.
-
SponsoredSmart completions using autonomous outflow control devices significantly helped in improving reservoir management and increasing oil and gas field productivity by enhancing the injection wells' performance.
-
SponsoredWith more surface facilities and infrastructure in oil and gas fields, well casing integrity is becoming an even bigger challenge. This article sheds light on the optimum way to deal with the increasing casing integrity challenges in the Middle East through field monitoring and time-lapse casing-integrity and corrosion-inspection logging.
Technology Focus
Recommended for You (Login Required for Personalization)
-
Texas has become an early hot spot for geothermal energy exploration as scores of former oil industry workers and executives are taking their knowledge to a new energy source.
-
Ignis H2 Energy and Imeco Inter Sarana announced a strategic partnership to expedite geothermal development in Indonesia.
-
One area where the untapped potential of geothermal energy has been realized is in harnessing thermal energy from abandoned or inactive oil and gas wells. Such wells could be exploited for electricity generation or direct use through well repurposing.
Content by Discipline